derivative
Time derivative of UAV states
Description
stateDerivative = derivative(uavGuidanceModel,state,control,environment)ode45 to simulate the UAV.
Examples
This example shows how to use the multirotor guidance model to simulate the change in state of a UAV due to a command input.
Create the multirotor guidance model.
model = multirotor;
Create a state structure. Specify the location in world coordinates.
s = state(model); s(1:3) = [3;2;1];
Specify a control command, u, that specified the roll and thrust of the multirotor.
u = control(model); u.Roll = pi/12; u.Thrust = 1;
Create a default environment without wind.
e = environment(model);
Compute the time derivative of the state given the current state, control command, and environment.
sdot = derivative(model,s,u,e);
Simulate the UAV state using ode45 integration. The y field outputs the multirotor UAV states as a 13-by-n matrix.
simOut = ode45(@(~,x)derivative(model,x,u,e), [0 3], s); size(simOut.y)
ans = 1×2
13 3536
Plot the change in roll angle based on the simulation output. The roll angle (the X Euler angle) is the 9th row of the simOut.y output.
plot(simOut.y(9,:))

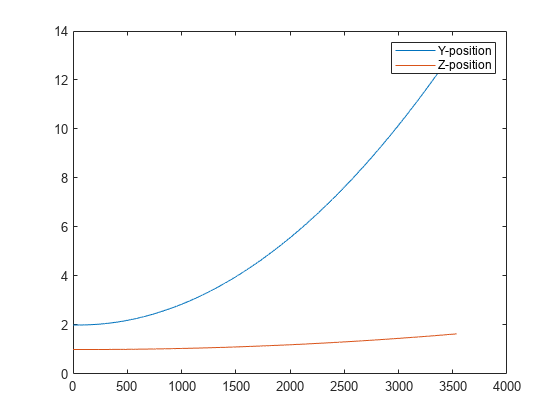
Plot the change in the Y and Z positions. With the specified thrust and roll angle, the multirotor should fly over and lose some altitude. A positive value for Z is expected as positive Z is down.
figure plot(simOut.y(2,:)); hold on plot(simOut.y(3,:)); legend('Y-position','Z-position') hold off
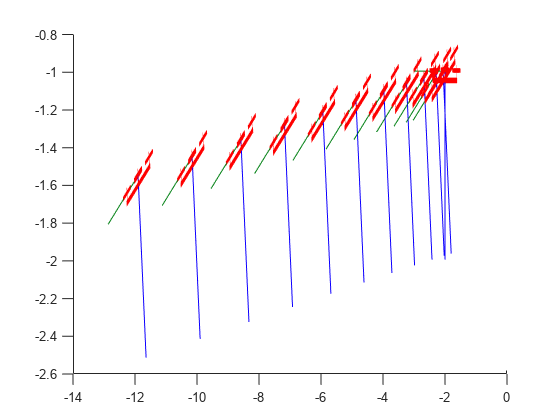
You can also plot the multirotor trajectory using plotTransforms. Create the translation and rotation vectors from the simulated state. Downsample (every 300th element) and transpose the simOut elements, and convert the Euler angles to quaternions. Specify the mesh as the multirotor.stl file and the positive Z-direction as "down". The displayed view shows the UAV translating in the Y-direction and losing altitude.
translations = simOut.y(1:3,1:300:end)'; % xyz position rotations = eul2quat(simOut.y(7:9,1:300:end)'); % ZYX Euler plotTransforms(translations,rotations,... 'MeshFilePath','multirotor.stl','InertialZDirection',"down") view([90.00 -0.60])
This example shows how to use the fixedwing guidance model to simulate the change in state of a UAV due to a command input.
Create the fixed-wing guidance model.
model = fixedwing;
Set the air speed of the vehicle by modifying the structure from the state function.
s = state(model);
s(4) = 5; % 5 m/sSpecify a control command, u, that maintains the air speed and gives a roll angle of pi/12.
u = control(model); u.RollAngle = pi/12; u.AirSpeed = 5;
Create a default environment without wind.
e = environment(model);
Compute the time derivative of the state given the current state, control command, and environment.
sdot = derivative(model,s,u,e);
Simulate the UAV state using ode45 integration. The y field outputs the fixed-wing UAV states based on this simulation.
simOut = ode45(@(~,x)derivative(model,x,u,e), [0 50], s); size(simOut.y)
ans = 1×2
8 904
Plot the change in roll angle based on the simulation output. The roll angle is the 7th row of the simOut.y output.
plot(simOut.y(7,:))

You can also plot the fixed-wing trajectory using plotTransforms. Create the translation and rotation vectors from the simulated state. Downsample (every 30th element) and transpose the simOut elements, and convert the Euler angles to quaternions. Specify the mesh as the fixedwing.stl file and the positive Z-direction as "down". The displayed view shows the UAV making a constant turn based on the constant roll angle.
downsample = 1:30:size(simOut.y,2); translations = simOut.y(1:3,downsample)'; % xyz-position rotations = eul2quat([simOut.y(5,downsample)',simOut.y(6,downsample)',simOut.y(7,downsample)']); % ZYX Euler plotTransforms(translations,rotations,... 'MeshFilePath','fixedwing.stl','InertialZDirection',"down") hold on plot3(simOut.y(1,:),-simOut.y(2,:),simOut.y(3,:),'--b') % full path xlim([-10.0 10.0]) ylim([-20.0 5.0]) zlim([-0.5 4.00]) view([-45 90]) hold off

Input Arguments
UAV guidance model, specified as a fixedwing
or multirotor
object.
State vector, specified as a eight-element or thirteen-element vector. The vector is always filled with zeros. Use this function to ensure you have the proper size for your state vector.
For fixed-wing UAVs, the state is an eight-element vector:
North - Position in north direction in meters.
East - Position in east direction in meters.
Height - Height above ground in meters.
AirSpeed - Speed relative to wind in meters per second.
HeadingAngle - Angle between ground velocity and north direction in radians.
FlightPathAngle - Angle between ground velocity and north-east plane in radians.
RollAngle - Angle of rotation along body x-axis in radians per second.
RollAngleRate - Angular velocity of rotation along body x-axis in radians per second.
For multirotor UAVs, the state is a thirteen-element vector in this order:
World Position -
[x y z]in meters.World Velocity -
[vx vy vz]in meters per second.Euler Angles (ZYX) -
[psi theta phi]in radians.Body Angular Rates -
[p q r]in radians per second.Thrust -
Fin Newtons.
Environmental input parameters, returned as a structure. To generate this structure,
use environment.
For fixed-wing UAVs, the fields of the structure are WindNorth,
WindEast,WindDown, and
Gravity. Wind speeds are in meters per second, and negative speeds
point in the opposite direction. Gravity is in meters per second squared (default
9.81).
For multirotor UAVs, the only element of the structure is Gravity
(default 9.81) in meters per second squared.
Control commands for UAV, specified as a structure. To generate this structure, use
control.
For multirotor UAVs, the guidance model is approximated as separate PD controllers for each command. The elements of the structure are control commands:
Roll- Roll angle in radians.Pitch- Pitch angle in radians.YawRate- Yaw rate in radians per second. (D = 0. P only controller)Thrust- Vertical thrust of the UAV in Newtons. (D = 0. P only controller)
For fixed-wing UAVs, the model assumes the UAV is flying under the coordinated-turn condition. The Guidance Model equations assume zero side-slip. The elements of the bus are:
Height- Altitude above the ground in meters.Airspeed- UAV speed relative to wind in meters per second.RollAngle- Roll angle along body forward axis in radians. Because of the coordinated-turn condition, the heading angular rate is based on the roll angle.
Output Arguments
Time derivative of state, returned as a vector. The time derivative vector has the
same length as the input state.
Extended Capabilities
C/C++ Code Generation
Generate C and C++ code using MATLAB® Coder™.
Version History
Introduced in R2018b
MATLAB Command
You clicked a link that corresponds to this MATLAB command:
Run the command by entering it in the MATLAB Command Window. Web browsers do not support MATLAB commands.
Sélectionner un site web
Choisissez un site web pour accéder au contenu traduit dans votre langue (lorsqu'il est disponible) et voir les événements et les offres locales. D’après votre position, nous vous recommandons de sélectionner la région suivante : .
Vous pouvez également sélectionner un site web dans la liste suivante :
Comment optimiser les performances du site
Pour optimiser les performances du site, sélectionnez la région Chine (en chinois ou en anglais). Les sites de MathWorks pour les autres pays ne sont pas optimisés pour les visites provenant de votre région.
Amériques
- América Latina (Español)
- Canada (English)
- United States (English)
Europe
- Belgium (English)
- Denmark (English)
- Deutschland (Deutsch)
- España (Español)
- Finland (English)
- France (Français)
- Ireland (English)
- Italia (Italiano)
- Luxembourg (English)
- Netherlands (English)
- Norway (English)
- Österreich (Deutsch)
- Portugal (English)
- Sweden (English)
- Switzerland
- United Kingdom (English)